12
$\begingroup$

Standard definitions state that a convex polyhedron is one where any line segment connecting two points within or on the polyhedron lies entirely within or on the polyhedron.

I'm curious if this definition can be simplified when dealing specifically with polyhedra:

  • Can we define a convex polyhedron by the condition that any line segment connecting two of its vertices lies entirely within or on the polyhedron?
  • Is there a non-convex (concave) polyhedron where all line segments connecting its vertices lie entirely within or on the polyhedron?

In other words, does considering only the vertices (and the line segments between them) suffice to determine the convexity of a polyhedron, or is it necessary to consider all points within the polyhedron?

$\endgroup$
3
  • 1
    $\begingroup$ Given two vertices A, B, how do you propose to test whether the whole of line segment AB lies in the interior or surface of the polyhedron? You'd have to know where that interior is, right? $\endgroup$
    – Rosie F
    Commented Nov 6 at 16:44
  • $\begingroup$ At an extreme one could have a figure (in 3D) consisting only of the edges between all vertices, such as the "skeleton" of a tetrahedron. This is something of the limiting case of the Answers posted below, although it doesn't have any interior and would not be considered a "polyhedron". $\endgroup$
    – hardmath
    Commented Nov 7 at 14:21
  • $\begingroup$ To add on to the already given answers, the answer to the question in the title is probably yes. However, the relevant condition one has to test for is that at each vertex, all directions joining it with other vertices lie in a common half-space. (It's not immediately obvious to me right now whether the condition of all these lines lying on the polyhedron is implied by this - if not, that is required in addition) $\endgroup$
    – Jan Nienhaus
    Commented Nov 8 at 2:41

2 Answers 2

Reset to default
21
$\begingroup$

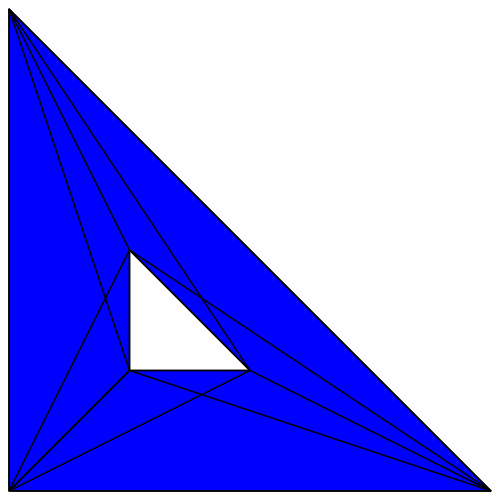
Consider a "hollow prism" whose cross-section is shown here: enter image description here

$\endgroup$
2
  • $\begingroup$ What if we eliminate the option to have holes (require the boundary to be connected)? $\endgroup$
    – R.. GitHub STOP HELPING ICE
    Commented Nov 8 at 1:20
  • $\begingroup$ We have this case already. $\endgroup$
    – Oscar Lanzi
    Commented Nov 8 at 9:15
17
$\begingroup$

An example with Euler characteristic 2 and simply connected faces, matching convex polyhedra:

Start with a tetrahedron. Drill a triangular hole into it, tapering to a point within the body of the original tetrahedron. The view from the direction of the removed vertex is given below; the hole is indicated by the light blue faces. (Yes, I wanted to make the hole regular, but lack of sufficient drawing options on my device forced me to compromise.)

enter image description here

The figure has seven faces, seven vertices, and twelve edges, thus Euler characteristic 2. With these vertex and edge counts there are nine diagonals; six of these are on the quadrangular faces and the remaining three run below the hole from the concave vertex to the basal ones.

In three dimensions a sufficient condition for convexity is to consider all connections between points on the edges of the polyhedron. In the example given here, all vertex-to-vertex connection lie on or inside the polyhedron; but a connection between the midpoints of two different boundaries of the shaded region (the hole) would pass over the hole and outside the polyhedron. Similarly segments between edges of the hole in Robert Israel's hollow prism can be drawn inside the hole and not in the polyhedron.

Another condition for convexity in three dimensions, which lends itself more readily to finite computation, involves the triangular regions defined by sets of three vertices. If all such regions lie on or inside the polyhedron, then the polyhedron is convex; but in both this example and Robert Israel's there are clearly triangular regions that cover the holes and thus go outside the polyhedron proper.

$\endgroup$
5
  • $\begingroup$ But if the polyhedron is homeomorphic to a compact solid ball (a compact 3-disk), and all space diagonals (segments between polyhedron vertices) fall in/on the polyhedron, then we can conclude it is a convex polyhedron? $\endgroup$
    – Jeppe Stig Nielsen
    Commented Nov 7 at 9:06
  • 3
    $\begingroup$ An even simpler example would be a difference between tetrahedrons ABCD and ABCE, where E lies within ABCD. $\endgroup$
    – Litho
    Commented Nov 7 at 13:42
  • 3
    $\begingroup$ @JeppeStigNielsen Isn't that exactly what this counterexample disproves, as opposed to the accepted answer? $\endgroup$
    – Misha Lavrov
    Commented Nov 7 at 22:23
  • $\begingroup$ Technically, the posted question did not ask for homeomorphism with a sphere, so both answers are valid. But yes, this example does respect homeomorphism with a sphere. $\endgroup$
    – Oscar Lanzi
    Commented Nov 8 at 0:02
  • $\begingroup$ @MishaLavrov, Hah, I had not even understood the example. This one is homeomorphic to a ball, just like you say. $\endgroup$
    – Jeppe Stig Nielsen
    Commented Nov 9 at 18:43

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .